How to calculate the FIDE Percentage Expectancy Table

Question

Section 8 of the FIDE Rating Regulations provides the following table

I understand that dp=800 and dp=-800 are arbitary caps at the top and bottom of the table.

However, in relation to the other scores, what's the formula for the conversion of p into dp, and vice-versa?

As noted in the comments, the regular Elo formula almost works but does not quite work. Does anyone know what the formula is for the adjustment made in the tails?

Answer 1

It's basically just table 8.1a with the columns switched. E.g. the entry in table 8.1b with rating difference 92-98 and expected score (for the higher rated player) of .63, corresponds with the entry (.63, 95) in table 8.1a.

That table is based on the expected score formula mentioned here:

If Player A has a rating of R_A and Player B a rating of R_B, the exact formula (using the logistic curve) for the expected score of Player A is

E_A = 1 / (1 + 10 ^{(R_B - R_A) / 400})

but it's also possible to directly generate table 8.1b from this formula. For example, a rating difference of 92 points (in A's favor) leads to E_A = 0.6294.

The inverse formula, calculating the rating difference which corresponds to a certain expected score, is R_B - R_A = 400 log₁₀(1 / E_A - 1).

Answer 2

As stated in 12.1 the formula above gives a close approximation to tables 8.1a and 8.1b. However it does not explain the precise construction of the table. Take fore example Rb-Ra = -736. According to the table 8.1b, the resulting expectation equals 100%. But according to the formula, the expectation is 98,57%.

Answer 3

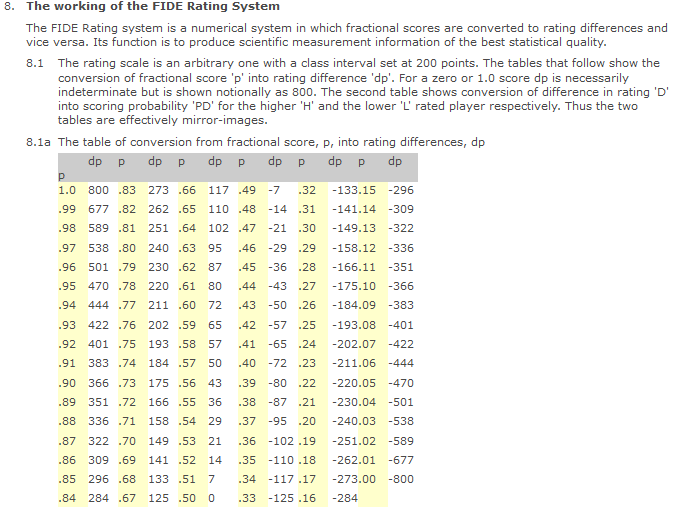
Table 8.1a is a mirror image of:

The Percentage Expectancy Table (A.E. Elo The Rating of Chess Players, 2.11)

   D        P    |    D       P    |    D        P
Rtg.Dif   H   L  | Rtg Dif  H   L  | Rtg Dif   H   L   
  0-3    .50 .50 | 122-129 .67 .33 | 279-290  .84 .16 
  4-10   .51 .49 | 130-137 .68 .32 | 291-302  .85 .15  
 11-17   .52 .48 | 138-145 .69 .31 | 303-315  .86 .14  
 18-25   .53 .47 | 146-153 .70 .30 | 316-328  .87 .13  
 
 26-32   .54 .46 | 154-162 .71 .29 | 329-344  .88 .12    
 33-39   .55 .45 | 163-170 .72 .28 | 345-357  .89 .11   
 40-46   .56 .44 | 171-179 .73 .27 | 358-374  .90 .10   
 47-53   .57 .43 | 180-188 .74 .26 | 375-391  .91 .09
 
 54-61   .58 .42 | 189-197 .75 .25 | 392-411  .92 .08   
 62-68   .59 .41 | 198-206 .76 .24 | 412-432  .93 .07    
 69-76   .60 .40 | 207-215 .77 .23 | 433-456  .94 .06    
 77-83   .61 .39 | 216-225 .78 .22 | 457-484  .95 .05 
 
 84-91   .62 .38 | 226-235 .79 .21 | 485-517  .96 .04    
 92-98   .63 .37 | 236-245 .80 .20 | 518-559  .97 .03 
 99-106  .64 .36 | 246-256 .81 .19 | 560-619  .98 .02
 107-113 .65 .35 | 257-267 .82 .18 | 620-735  .99 .01
 114-121 .66 .34 | 268-278 .83 .17 | over735 1.00 .00

How to reproduce this table?

Since the normal curve flattens, one would expect that the number of rating differences does not decrease. However, there is a discontinuity at D = 345-357, P = 89%. This suggests that the table may contain irregularities

Rtg Dif Range                
279 290 11
291 302 11  
303 315 12
316 328 12
329 344 15
345 357 12 <---
358 374 16
375 391 16  
392 411 19

Construction of the table is most likely:

• Let sigma = 200 * (10 / 7), where 10 / 7 is an approximation for sqrt(2). Reference: A.E. Elo, Theory of Rating Systems, privately printed monograph, 1966.
• Convert 0, 20, 40, 60, etc. into Z scores: 0, 0.07, 0.14, 0.21, etc. using Z = D / sigma. The corresponding probabilities can be found in the "Standard normal table", which gives 0.5000, 0.5040, 0.5080, 0.5120. Elo could have used table X, p.91 from Garrett, H.E. Statistics in Psychology and Education. David McKay , New York , 1966.
• Intermediate values can be derived by linear interpolation.

The following differences exist between this calculation (in R base) and the Elo table:

D     <- c(343, 344, 358, 392)      # import from Elo table                      
P     <- c(.88, .88, .88, .92)      # import from Elo table 
dD    <- 20L                        # increment    in D = 0, 20, 40, etc.
sd    <- 200L * (10L / 7L)          # standard deviation.
dZ    <- 0.07                       # dD / sd, increment in Z.
Z0    <- trunc(D / dD) * dD / sd    # index in Z table begin.
Z1    <- round(Z0 + dZ, 2)          # index in Z table end.
PZ0   <- round(pnorm(Z0), 4)        # P before.
PZ1   <- round(pnorm(Z1), 4)        # P after.

ix    <- D %% dD                    # index 0 - 20.
Pstep <- (PZ1 - PZ0) / dD           # interpolation step.

P4    <- PZ0 + ix * Pstep           # intermediate percentage.
P2    <- round(P4 + 5E-5 + 1E-6, 2) # rounded up to 4 decimals then 2.
Diff  <- round(P2 - P, 15)          # Diff with Elo table.
cbind(D=D, P=P, Diff=Diff, P2=P2, P4=P4, Pstep=Pstep, ix=ix, PZ1=PZ1, PZ0=PZ0, Z1=Z1, Z0=Z0)

Output.

##        D    P  Diff   P2      P4   Pstep ix    PZ1    PZ0   Z1   Z0
## [1,] 343 0.88  0.01 0.89 0.88498 0.00066  3 0.8962 0.8830 1.26 1.19
## [2,] 344 0.88  0.01 0.89 0.88564 0.00066  4 0.8962 0.8830 1.26 1.19
## [3,] 358 0.88  0.01 0.89 0.89488 0.00066 18 0.8962 0.8830 1.26 1.19
## [4,] 392 0.92 -0.01 0.91 0.91480 0.00055 12 0.9192 0.9082 1.40 1.33

Elo probably made a few clerical errors during the laborious manual calculation.

##      D    P   Diff   P2      P4    Pstep  ix    PZ1     PZ0    Z1   Z0
##     343 0.88     0 0.88 0.88416 0.000720*  3 0.8962  0.8820* 1.26 1.19
##     344 0.88     0 0.88 0.88488 0.000720*  4 0.8962  0.8820* 1.26 1.19
##     358 0.90     0 0.90 0.89496 0.000720* 18 0.8962  0.8820* 1.26 1.19
##     392 0.92     0 0.92 0.91498 0.000565  12 0.9195* 0.9082  1.40 1.33