Some statistics indicate white scores 52-56% - but I wonder if that differs according to playing style - e.g. perhaps strong defenders prefer the black pieces.
Do any former/current grandmasters have stronger win records with the black pieces than with white?
Note: the reason for choosing only grandmasters in this question is simply to ensure a large number of games had been recorded for the player so as to exclude any player who only played a small number of games
Yes, they do, although very few GMs do and by a margin of less than 10%. This does not seem to be due only to random factors (see long answer).
An example is GM Joseph Gallagher. As you can see in his FIDE profile page, he has the following games record (as for 15 Dec. 2020):
White +124 =118 -73, score = 58.1%
Black +126 =124 -67, score = 59.3%
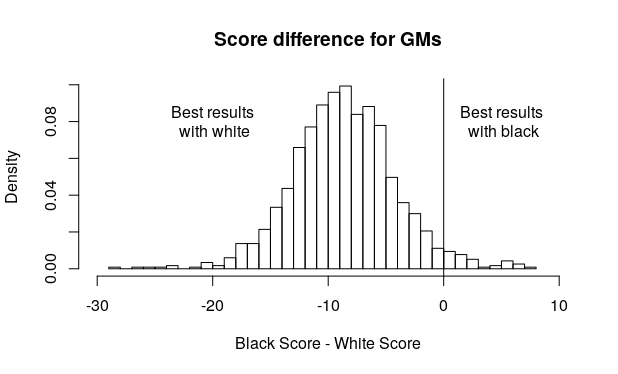
To obtain the figure above I used the games published by TWIC, issues 920-1358 (25 Jun. 2012 to 16 Nov. 2020). I selected games where at least one of the players was a GM. And I kept only players with more than 50 games per color in the dataset. This ensures that scores are calculated with a 90% confidence interval of at least ±2%, and, since players in the dataset have in average a couple of hundred games, scores are usually still more accurate. The resulting dataset had 561K games for 1168 GM players.
In this case, the average scores where 65.5% for white and 56.9% for black. This is higher than in the databases you mention because being a GM most other players have a lower rating and they're more likely to win. (If I took only the games where both players were GMs, the average scores were 52.9% for white and 43.3% for black.)
As shown in the figure above, the average difference of scores between both colors is 8.6%, favorable to white. Still, there were 38 players who had better results overall with black. The five players with the highest difference in performance favorable to black in the dataset were:
WhiteScore BlackScore Difference
Bryan Smith 55.2 62.9 7.8
Essam El Gindy 52.0 58.8 6.9
Jean-Luc Chabanon 50.5 57.2 6.7
Justin Tan 54.5 61.2 6.7
Mihai Suba 57.9 63.8 5.9
I would like to bring your attention to one of these players, Mihai Suba, who is known for his book Dynamic Chess Strategy. In it he disagrees with white's first move advantage theory.
Note: If you look at these players' FIDE profile pages, you might find some discrepancies, due to the different characteristics of both datasets. The FIDE data includes all FIDE rated games (whether the player was already a GM or not); while my dataset contains games published on TWIC (FIDE rated or not) from 2012 to 2020 only if the player was already a GM.
Now, just because of randomness we would expect that some players had better results with black than with white. But is it the case? Do those players really perform better with black pieces or is it simply a random illusion? To check this I plotted the evolution of the score difference as the number of games increases for several players.
As you can see, the score difference varies widely when there are few games. But as the number of games increases, the variability decreases. In some cases, the score difference remains consistently above 0. In other cases, it occasionally crosses the line.
So, yes, there are some cases that cannot be considered a statistical artifact.
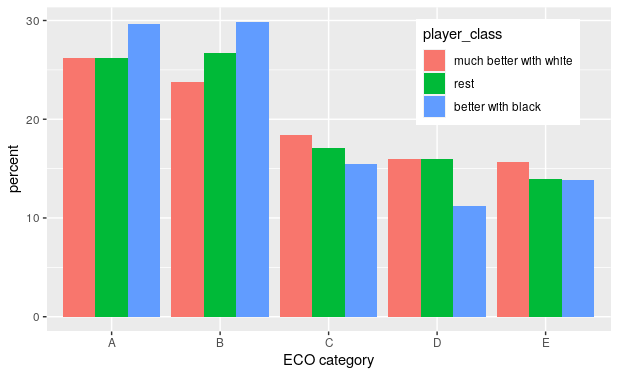
You didn't really ask about it, but since you mentioned it in your question I had a look at it. For this I divided the players in three groups according to their score difference:
And then I looked which kinds of openings they used, classified according to the five main ECO categories:
It would seem that GMs who have better results with black pieces tend to use openings of the groups A and B more often than the other players, and the other groups less often. Although more detailed analyses would be necessary to arrive at more solid conclusions, this can give you some food for thought.
Following the request by @stevec, I have shared the dataset and script used to write this answer on github. It can be used to investigate some of the proposals made by other users (@HaukeReddmann, @SecretAgentMan) in the comments.
@Grade'Eh'Bacon, suggested in a comment comparing the observed distribution of the difference in scores to the expected distribution under only random influences. I had already done this analysis, but I decided not to include it because the answer was rather long. But since it has been requested, I share it here.
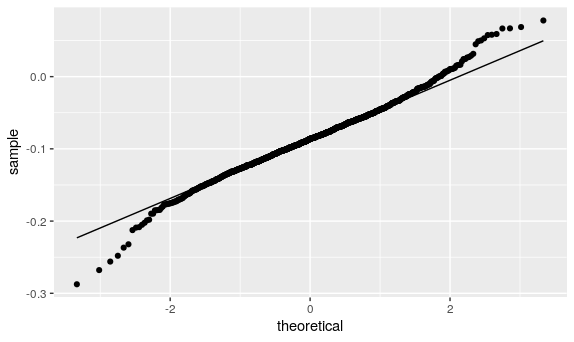
If the distribution shown in the score differences plot at the top of the answer was due only to random factors, we would expect a normal distribution (aka Gaussian distribution, bell curve). This is the hypothesis assumed in Elo calculations and can be checked with a Monte Carlo simulation.
One way to test if a variable follows a normal distribution is with a Q-Q plot (see below). If the observed score difference followed a normal distribution, the points would be close to the diagonal line. This happens in the middle zone, but not in the extremes. We have a distribution with heavy tails, that is, there are too many players with better results with black and too many players with much better results with white to be due to random causes alone.
The results of the Shapiro-Wilks test, which is also used to test for normality is also consistent with the hypothesis that the distribution is not normal. This supports the hypothesis that there is something else going on apart from randomness.
And I also repeated the analysis with another independent dataset of games from the ICCF. And I obtained comparable results.
White scores about 54%, which is quite different from White winning 54% of all game, more so considering how draws occur more often between stronger players.
None of the top players score better as Black, and I doubt there are grandmasters who do. If that were the case, it'd definitely be due to a small sample. I used to have a better score as Black back when I was an 1800-rated player, but it soon got corrected.
