KBN vs K checkmate on nonstandard boards

Question

I know how to win the endgame with bishop and knight, but it is a slippery process and seems to be just barely a win, with the enemy king very nearly escaping. For this reason, I am curious about this endgame on other board sizes and if it will still be possible in the general case of an MxN board. Let's assume the 50 move rule doesn't apply.

Here are a few examples.

• Is there a forced win on a 10x10 board?
• Is there a forced win on a 7x7 board, with a bishop of the "wrong"' color (i.e. a bishop that can't attack the corner squares)?

Answer 1

Actually, the bishop and knight mate is not as slippery as it appears. I have checked this on a tablebase program I wrote. On a 10x10 board, the side with the bishop and knight (say white) can force mate in at most 47 moves. White can even force mate on a 16x16 board, in at most 93 moves. I believe mate can be forced on an arbitrarily large even size board.

First, on an odd size board, I've confirmed that white cannot force mate if the bishop is on the wrong color. Mate can only be forced in a good corner (one that the bishop controls), so if there are no good corners, mate cannot be forced.

On the 10x10 board, the following is an optimal mate in 47. The starting position is W: Ka1, Nb1, Bc1; B: Kc2. 1.Bb2 Kb3 2.Ba3 Kc2 3.Ka2 Kd3 4.Kb3 Ke4 5.Kc4 Ke5 6.Bg9 Kf4 7.Kd5 Kf5 8.Be7 Kf4 9.Ke6 Kg4 10.Ke5 Kf3 11.Kf5 Kg2 12.Kg4 Kf2 13.Kf4 Kg2 14.Nd2 Kh1 15.Kg3 Ki2 16.Nf3 Ki1 17.Kh3 Kh1 18.Bf6 Ki1 19.Nh2 Kh1 20.Bj2 Kg1 21.Ng4 Kf1 22.Kg3 Ke2 23.Nf2 Kd2 24.Bf6 Ke3 25.Bg7 Kd2 26.Kf4 Kc2 27.Ke4 Kd2 28.Bd4 Ke1 29.Nh1 Kf1 30.Kf3 Ke1 31.Be3 Kd1 32.Ke4 Kc2 33.Kd4 Kd1 34.Kd3 Ke1 35.Ng3 Kd1 36.Bc5 Ke1 37.Bd4 Kd1 38.Bc3 Kc1 39.Nf5 Kd1 40.Ne3 Kc1 41.Kc4 Kb1 42.Kb3 Kc1 43.Be1 Kb1 44.Bd2 Ka1 45.Nc2+ Kb1 46.Na3+ Ka1 47.Bc3#

This can be played out on apronus.com.

After 23. Nf2, we have a position just like the one shown in Andrew's answer (but upside down: W: Kg3, Bj2, Nf2; B: Ke2). If we make this board 8x8 by removing the a and b columns (and rows 9 and 10), it would be mate in 14, but here it's mate in 25. In the optimal line above, the black king never really tries to escape towards the a10 corner. Let's say he does, with 23. ... Kd2 24. Bf6 Kc2. This move shortens the mate by one move, with the continuation 25.Kf3 Kb3 26.Ke4 Ka4 27.Kd5 Kb5 28.Bd4 Ka4 29.Kc4 Ka5 30.Kc5 Ka6 31.Kc6.

The black king can only escape as far as a6, and is ultimately still trapped in the good a1 corner. The rest of this continuation is 31. ... Ka5 32.Nd3 Ka4 33.Kc5 Ka5 34.Nb4 Ka4 35.Kc4 Ka5 36.Be3 Ka4 37.Bb6 Ka3 38.Nd3 Ka4 39.Nb2 Ka3 40.Kc3 Ka2 41.Kc2 Ka3 42.Ba5 Ka2 43.Bb4 Ka1 44.Nd3+ Ka2 45.Nc1+ Ka1 46.Bc3#

Here is the number of moves to force mate on every even sized board from 4 to 16. 4: 15; 6: 22; 8: 33; 10: 47; 12: 64; 14: 78; 16: 93. Note that on any size board there are a handful of positions that are drawn because black can win a piece immediately.

The following is an optimal mate in 92 on a 16x16 board. The starting position is again W: Ka1, Nb1, Bc1; B: Kc2. 1.Bb2 Kb3 2.Bi9 Ka4 3.Kb2 Kb5 4.Kc3 Kc6 5.Kd4 Kd7 6.Ke5 Ke8 7.Kf6 Kf8 8.Kg6 Kg8 9.Bg11 Kf9 10.Kh7 Ke10 11.Kg8 Kf11 12.Bi9 Ke10 13.Kh9 Kd11 14.Kg10 Ke10 15.Bg11 Kd9 16.Kf9 Kc10 17.Ke10 Kc11 18.Ke11 Kc12 19.Nd2 Kd13 20.Ne4 Ke14 21.Nf6 Kf13 22.Kf11 Ke14 23.Ke12 Kd15 24.Kd13 Ke16 25.Ke14 Kd16 26.Nd7 Kc16 27.Ne9 Kb15 28.Kd15 Kb14 29.Bf10+ Kb15 30.Nd11 Ka16 31.Nc13 Kb16 32.Kd16 Ka15 33.Kc15 Ka16 34.Kc16 Ka15 35.Na12+ Ka16 36.Nb14 Ka15 37.Nd13 Ka14 38.Nc11 Ka13 39.Bc13 Ka14 40.Kc15 Ka13 41.Kc14 Ka14 42.Bd12 Ka13 43.Na10 Ka12 44.Kc13 Kb11 45.Nb12 Ka12 46.Kc12 Ka13 47.Be11 Ka12 48.Bf12 Ka13 49.Bc15 Ka12 50.Nd11 Ka11 51.Bf12 Ka12 52.Nc13 Ka11 53.Kc11 Ka10 54.Nd11 Ka9 55.Nb10 Kb9 56.Kb11 Ka9 57.Kc10 Ka10 58.Bg13 Ka11 59.Be15 Ka10 60.Nd9 Ka9 61.Bh12 Ka10 62.Nc11+ Ka9 63.Kc9 Ka8 64.Nd9 Kb7 65.Nb8 Ka7 66.Kc8 Ka8 67.Bg11 Ka9 68.Be13+ Ka8 69.Nd7 Ka7 70.Bh10 Ka8 71.Nc9 Ka7 72.Kc7 Ka6 73.Kc6 Ka7 74.Bd6 Ka6 75.Bc5 Ka5 76.Ne8 Ka4 77.Kd5 Kb3 78.Kd4 Kc2 79.Bb4 Kb3 80.Kc5 Ka2 81.Kc4 Kb1 82.Kc3 Kc1 83.Nd6 Kd1 84.Kd3 Kc1 85.Nc4 Kd1 86.Ba5 Kc1 87.Bd2 Kb1 88.Kc3 Ka2 89.Kc2 Ka1 90.Kb3 Kb1 91.Na3+ Ka1 92.Bc3#

It is long, but playing through it definitely helped convince me that white could force mate on an arbitrarily large board. In the first phase, the white king and bishop can corral the black king while buying tempi for the white knight to catch up. Once the black king is trapped in the bad corner (a16 in this case), it is shuffled down the a-file with very little breathing room. Although the procedure is significantly more complicated than a W maneuver, white appears to always be in complete control.

Answer 2

Let's start with the 7x7 question:

Is there a forced win on a 7x7 board, with a bishop of the 'wrong' color?

This seems to be the easier of the two questions to answer. First, convince yourself that this is the only mating pattern (the Black king could also be on the dark square immediately to its left).

[FEN "8/8/8/8/8/4B1NK/8/7k w - - 0 1"]

The key point is that it is not possible for White to force this position. Black's king would have been stalemated on the previous move. Alternatively, if Black's king is moved one square to the left, the only legal move that white could have just played would be to move the bishop onto that diagonal, delivering mate. If this were the case, where was Black's king before that? It would have been on f2 (two to the left, one up). So Black was not forced to move into the corner and could have instead avoided the mate. To conclude, there is no way to force mate in the wrong corner; shortening the board doesn't change this fact.

Now the first question:

Is there a forced win on a 10x10 board?

In this case, White will have a proper corner, but let's assume that White can force the Black king into the wrong corner. n the standard 8x8 board, White has to release the king from the side for a few moves in the process of driving the king to the mating corner. See Wikipedia for a complete tutorial. Here is the normal position when Black flees the edge (temporarily).

[FEN "8/2kN3B/4K3/8/8/8/8/8 w - - 0 1"]

Black usually plays Kc6, and then after Bd3!, the king has no escape. On a 10x10 board, however, Black could play Kb7, followed by Ka7, and finally, Kz6, as we will call the first file to the left "z". White has no way to get the king and knight over to help prevent the Black king from escaping the bind. So again, it's a good thing that the board is only 8x8, otherwise, the bishop and knight would never get to mate the king!

_{Disclaimer: I have not proven any of my assertions with tablebases}

Answer 3

There are obviously many forced wins on any boards where M and N are at least 8 (including M or N or both infinite), so long as there is a corner of the same colour as the bishop's square.

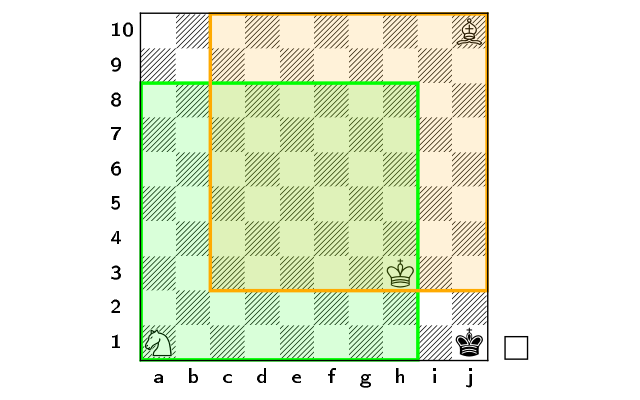
If the pieces are all in the yellow tinted sub-board and the black king cannot escape the d10-j4-j10 triangle, the position is also won on the full board, because such positions can be (optimally) won on that sub-board without letting the black king escape the triangle. Similar goes for the green sub-board. The same applies to an MxN board.

But the won positions are by no means restricted to such positions. In the position shown, for example, White can mate in at most 33 moves against any Black defence. There is, of course, a significant percentage of similar positions.

There are not necessarily forced wins if M and or N are too small. For example, there are no checkmate positions on a 1xN board.

There are, strictly speaking, also a relatively small number of forced wins on (sufficiently large, i.e. M,N > 2, M+N>6) boards which include no corner of the same colour as the bishop's square but do include a corner of the opposite colour. This includes the 7x7 board with the "wrong" coloured corners you ask about. This is also possible in a "wrong" corner of any board that includes such a corner, e.g. on an 8x8 board.

[FEN "7k/8/7K/8/5N2/8/8/7B w - - 0 1"]

1. Ng6+ Kg8 2. Bd5#**

There are no wins on a board that includes no corners, i.e. where one or both sides extend indefinitely in both directions.

There are drawn positions on any size of board. This is the general case on boards that have no corner of the same colour as the bishop's square and on boards where one or both of M and N are too small and, I believe, on boards where M and N are both large. Here is one example on an 8x8 board.

[FEN "8/8/8/8/3K2k1/8/1B6/7N b - - 0 1"]
[startflipped ""]

1... Kf3

Drawn positions are the exception on the standard board (less than 10% of all positions according to the Nalimov EGTB).

But I believe that on a 10x10 board there are also draws by repetition, where the lone king cannot force the capture of a piece, but the side with the pieces also cannot force mate. I think this becomes the general case for large M and N as it obviously is for odd M and N with the "wrong" coloured bishop.

So long as the board contains a corner of the same colour as the bishop's square and M or N remains at 8 or less, but it is not too small, mate will still be forcible generally for finite large values of the other and (somewhat irrelevantly) in as many positions as not for an infinite value of the other.

Edit:

After reading DanStronger's post, I think my comments on draws by repetition on larger boards are erroneous. These were based on a 45-year-old analysis that I made when I first learned to play the ending (the details of which are now hazy). But I'm inclined to think the analysis was flawed. In that case, the percentage of draws should actually decrease as the board sizes increase.

Answer 4

I think the biggest distinction we can make here is how many moves it will take to mate the king. There is plenty of evidence above that proves it is possible to mate on an almost ainfinitely increasing board (assuming it stays a square and not a rectangular, as to why ai have no idea). In a tournament there is a 50 move rule to prevent needlessly long games. It is possible to mate with this scenario on a 8x8 board within the confines of 50 moves but with little room for error. The bigger the board, the more space you need to corral the King into the corner m which results in 90+ move mates.

To summarize, as long as the board is square (Length=width), then a KBN vs K mate is achievable. I cannot answer if the board is rectangular. aid someone else knows, they can answer that if they want or you could edit you question!