3

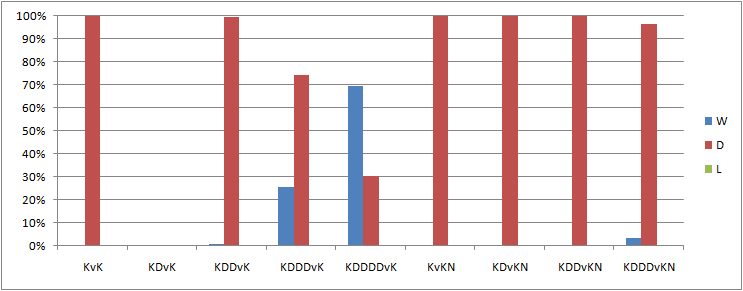
The dabbaba is a '2,0' leaper fairy piece, only able to ever reach a quarter of the squares on the board. According to the linked Wikipedia page, a quartet of them can apparently "easily" force mate against a bare king with the help of their own king; however, I'm curious whether an orthodox knight could reliably hold off the dabbaba quartet. It's effectively a 7-piece endgame, just that 4 of the pieces are weaker fairy pieces; four '2,0' leapers that can each only reach a quarter of the squares on the board versus a single '2,1' leaper that can reach any square on the board.

As for the dabbaba's aforementioned restriction to a quarter of the squares on the board, a single dabbaba can reach either ACEG or BDFH files and either odd ranks or even ranks; making the 4 dabbabas of the quartet be the odd ACEG, even ACEG, odd BDFH, and even BDFH. Assuming the 4 dabbabas are to cover their respective quarters of the board, there should be ((16^4)/8)*(60!/(60-3)!)*2 meaningfully unique KDDDDvKN positions to look at minus any where side-to-move could just take the other side's king or is already checkmated by the other side.

Improve this question
1
  • 1
    I'm currently making a simplified tb generator for 6x6 chessboard, only leapers, win by capturing the king, and no stalemates, as an excercise inspired by this post. I don't know if I will be able to do KDDDDvKN on my machine, but KDDDvKN is achievable. Will report later once I finish it.
    – Sopel
    Commented Jun 26, 2019 at 13:50

2 Answers 2

Reset to default
4

I was unable to generate data for exactly what you want, but I managed something with a restriction to 6x6 board. I think it's reasonable to assume that it doesn't make a big difference from 8x8 for this specific case since there are no powerful pieces on the board (with unrestricted movement distance).

Available positions are enumerated by starting from a certain position and doing moves until we can. It should give all possible positions but I'm not sure. (I respect the requirement posed by OP that all Ds have different available positions)

Code (C++17, uses a few MSVC specific intrinsics) (tuned for this specific piece types and configuration): https://pastebin.com/d6J5WHuY

Stripped WDL data:

        W               D               L
KvK     0.000000000%    100.000000000%  0.000000000%
KDvK    0.000000000%    0.000000000%    0.000000000%
KDDvK   0.620803000%    99.379200000%   0.000000000%
KDDDvK  25.666000000%   74.334000000%   0.000000000%
KDDDDvK 69.638300000%   30.361700000%   0.000000000%
KvKN    0.000000000%    100.000000000%  0.000000000%
KDvKN   0.000761905%    99.997700000%   0.001523810%
KDDvKN  0.083948400%    99.916000000%   0.000000000%
KDDDvKN 3.553240000%    96.446800000%   0.000000000%

enter image description here

        Longest DTM for white   
            Longest DTM for black
KvK     0   0
KDvK    0   0
KDDvK   17  0
KDDDvK  57  0
KDDDDvK 63  0
KvKN    0   0
KDvKN   1   1
KDDvKN  17  0
KDDDvKN 63  0

enter image description here

I'm unable to generate KDDDDvKN as it would require ~32GiB of RAM (should take less than an hour though), which I don't have.

Buf overall it can be seen that around a quarter of KDDDvK games are winning for white which may suggest that there are non-trivial winning KDDDDvKN positions.

I also have more detailed logs with the longest winning line shown. https://drive.google.com/drive/folders/1_aLMYB87zVJ9qslQxafTZNKHgXsoBnl2?usp=sharing

I have not added any persistence/querying and I don't plan to. Code is provided above.

Improve this answer
3
  • 1
    That's very nice, but I think you are providing the right answer to a wrong question. I am pretty sure any strong chess player can answer without writing a single line of code. I'll try to review the endgame this evening
    – David
    Commented Jul 2, 2019 at 7:39
  • 2
    It's intriguing to me that KDDDvK has a 25% win rate; based on Laska's answer which made sense to me, I'd assumed that KDDDvK would have no forcing lines or only very short forcing lines. Yet there's apparently a line that lasts for 57 half-moves. I'll still hold out for stats on KDDDDvKN, but this is good~ @David, though my question specifies "endgame statistics"; a strong player offering intuition about why certain recognizable KDDDDvKN position types are won or drawn and how the wins and draws work in those position types is still also interesting to me. I'm looking forward to your analysis
    – RadiantDarkBlaze
    Commented Jul 5, 2019 at 17:03
  • Why does it say 0% 0% 0% for KDvK? I think it should say 0% 100% 0% or something like that.
    – Lucenaposition
    Commented Jun 22 at 0:45
1

Hi welcome to chess stackexchange. If I had a Knight surely I can trade it for one of these Daddaba guys. So a simpler question is whether KDDD can beat K. If the lone K can reach the corner where he can’t be checked, then he is safe.

Improve this answer
1
  • 2
    I'm asking this question exactly because I'm not as sure as you are that the KDDDD side would allow that trade quite so easily. While your simpler q-a is intuitively accurate, I believe you're simplifying the question a bit hastily on the assumption that a trade is possible to force. Can you offer a reason why the trade of the knight for one of the dabbabas might be impossible for the KDDDD side to prevent, please?
    – RadiantDarkBlaze
    Commented Jun 16, 2019 at 7:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.