Distribution of Elo ratings

Question

Danger! Only for math buffs!

As we all know, Elo is Gaussian. In theory. Except it isn't. But my question is not about that distribution. Your Elo might also list a second number: the number of tournaments rated. To my best knowledge, FIDE Elo only has the "K number". My German national Elo ("DWZ") lists this number, though. Can you say something about the statistical distribution of that variable, making some natural assumptions on the process (players take up and leave chess with a constant rate; the number of tournaments per time of individual players might be Gaussian)? I also would be interested in a numerical simulation of that process.

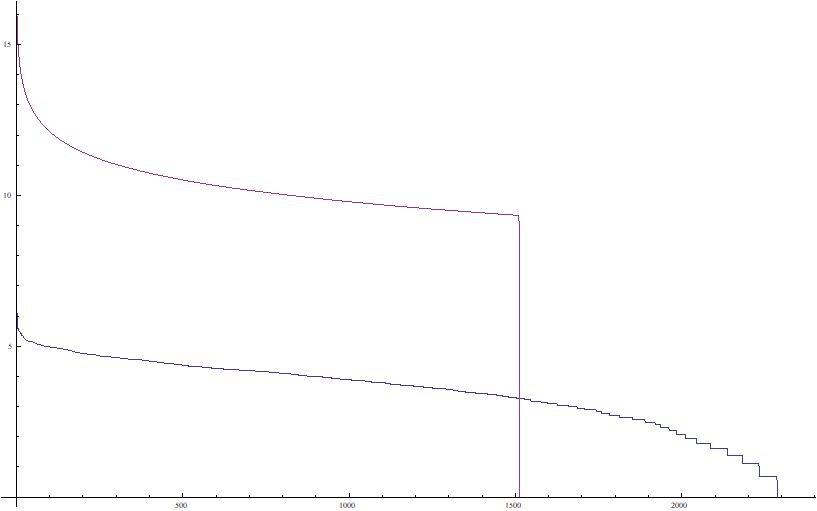
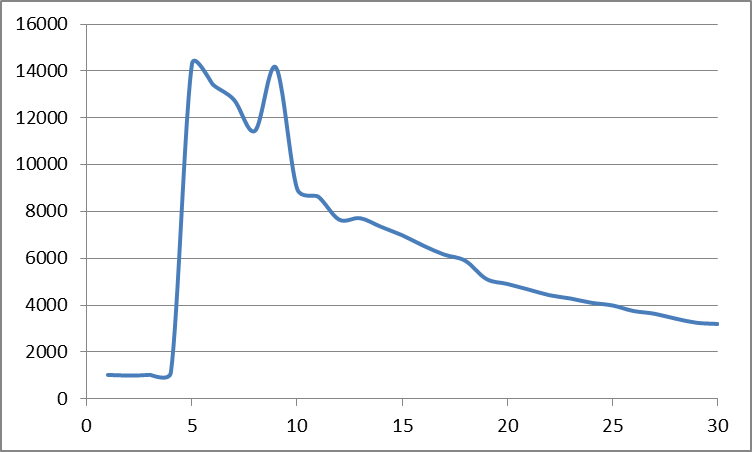
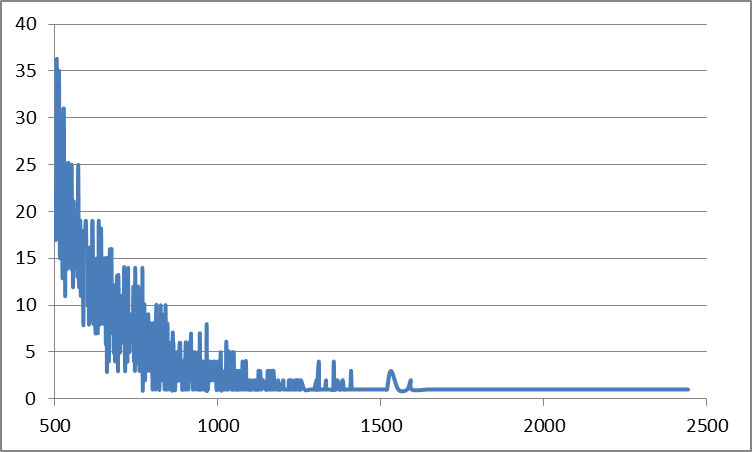
Legend: The lower curve was compiled by me and shows the number of rated tournaments of all rated Hamburg players, just pre-Corona. (I'm a chess maniac and show-off, I'm #2 or so :-) The upper curve - which determines the axis numbers! - is from my CS bachelor thesis and completely unrelated, but I found it always funny how similar it looks, except for the cutoff. Unfortunately I don't know a formula for that one, either. In first approximation, both are Poisson or Zipf or so.)

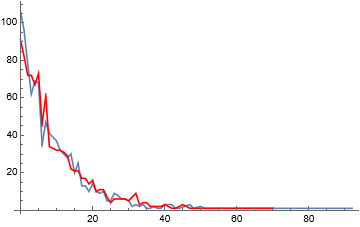
EDIT! As announced, here is the output of a very primitive simulation. We have an array A of length 1000 (players), with p=0.01 a chessplayer drops out and at the same time a new one starts at 0 (which is of course not realistic). With q=0.1, a player gets a new tournament rating (again, as stated in a comment, more than one should be updated). Essentially the only free variable is p/q (assuming both are smallish). Red: 50000 runs, Blue: 100000. (A player with 92 rated tournaments leads by far.) Evidently, a dynamic steady state was reached.

Answer 1

Your Elo might also list a second number: the number of tournaments rated. To my best knowledge, FIDE Elo only has the "K number".

Wrong.

The monthly rating lists which FIDE publishes include the number of games played which is a proxy for number of tournaments played and probably a more useful one than that.

Here is a graph of the number of players (y-axis) who played x number of games (x-axis) so far in 2022. These are FIDE rated at standard time control:

Not all that useful really because 276,251 have played zero games so far this year. Let's try again but remove that data point.

Now what we see is much more interesting. The maximum is 11,303 players playing exactly 5 games. To me that looks like a weekend 5-round Swiss in which all of your opponents are rated.

The little glitch comes at 9 games with 6886 players - 9 round all-play-all?

Let's extend the range to all FIDE rated standard control games played since 2010. The shape of the full graph isn't all that interesting so let's look at 1 through 30 games.

Local maxima at 5 games (14294 players) and 9 games (14149)!
Roughly 1000 players playing each of 1, 2, 3 or 4 rated games.
Over a 12 year period most of the players played just a handful of tournaments.

For balance let's look at the fanatics, those who played 500 or more games during the 12 years.

One player played 2442 games, about 200 games a year and 156 players played 1200 or more games.

Answer 2

I'd love to comment but alas, my reputation is too low. If this must be taken down, I understand. I suspect "the number of tournaments per time of individual players might be Gaussian" is not Gaussian as it is lower bounded at 0.

num. of tournaments is always positive. Per time of individual players is also positive. +/+ is always positive or zero.

Additional thoughts: In the contrived case where Hamburg players play only in Hamburg amongst themselves. I think the most interesting part here is how dependent each observation is. One player can not add another tournament appearance without adding tournament appearances for someone else. You can't play in a tournament by yourself after all. So it would be really neat to view this as a network where each player is a node and then tournaments are the lines connecting them.

• Edit: at this point, I think I have the karma to move this to the comments. But I don't wanna mess with things and maybe lose that privilege, so I will keep it here